Methods for Yaw Rate Control

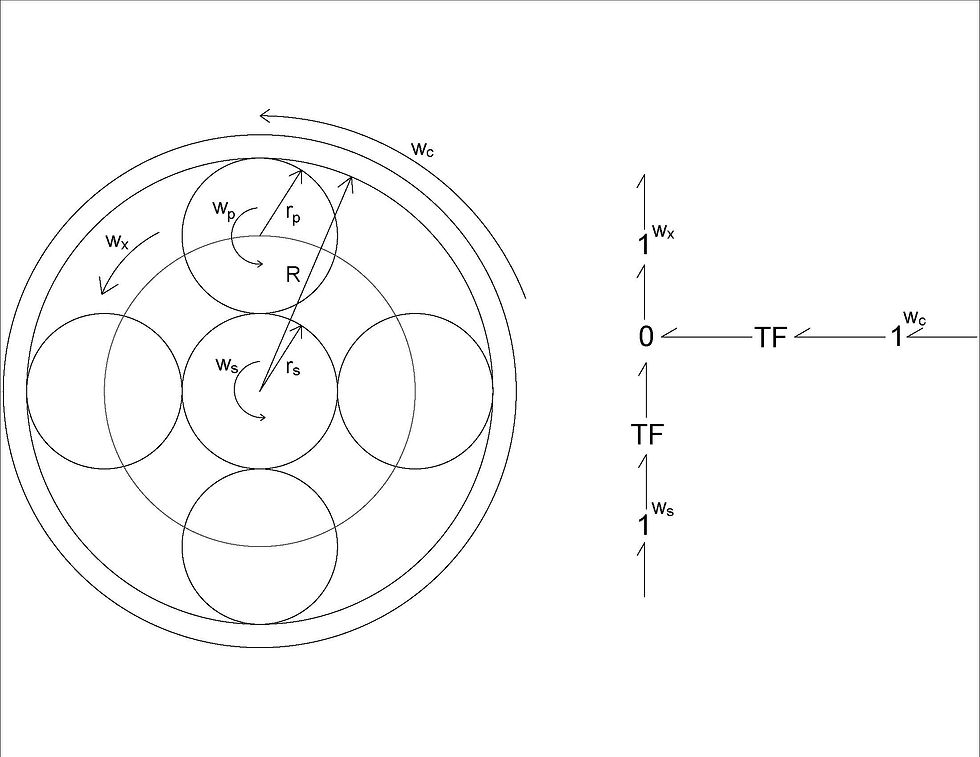
Additive steering using planetary gearbox is proposed to control the yaw rate errors between the 3-wheeler and the 4-wheeler. In anticipation of this method, in the figure it shows the schematic and bond graph fragment of the planetary gearbox for the additive front steering control system. The angular velocity w_s is the driver’s input,  w_c is the control input, and w_x is the resultant pinion gear angular velocity that steers the front wheels. When the controller is off, meaning w_c = 0, w_x is dependent purely on w_s. By using planetary gearbox for additive steering, the controlled steering input will not have any feedback to the driver's input, meaning the driver won't feel the controlled steering wheel is fighting with him/her. As it's obvious to tell, the w_s can be 0 while w_x can still be steered by w_c.

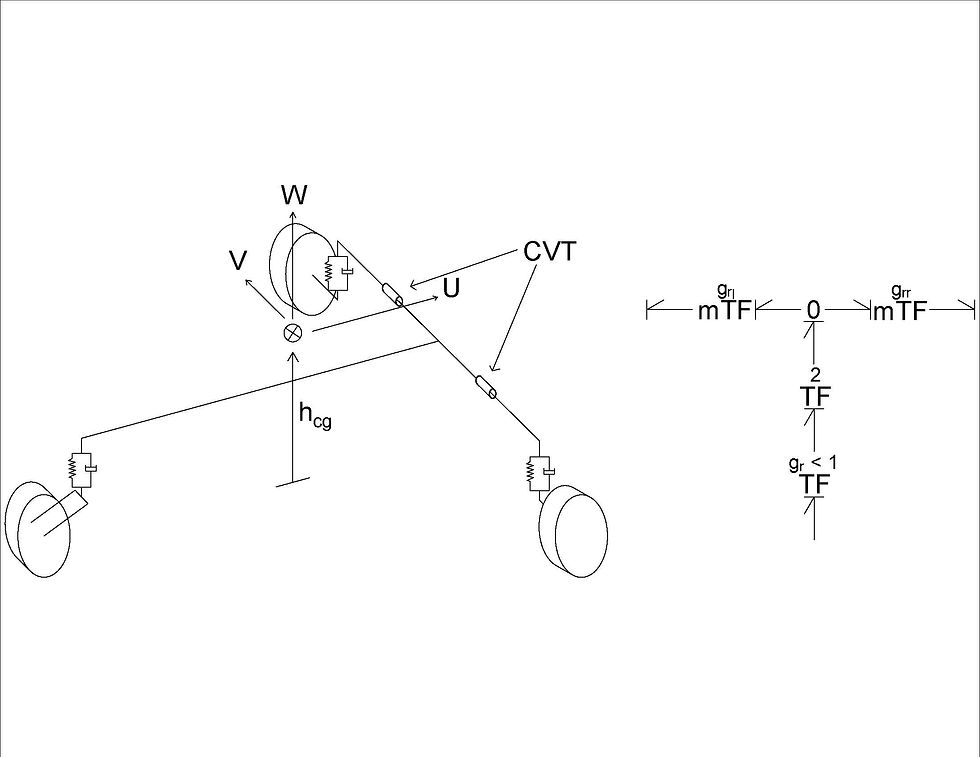
Another potential torque control concept is using CVT’s in the half shafts at the front of the 3-wheel vehicle. In anticipation of this control, Figure 8 shows the schematic and bond graph fragment of the CVT in the half shafts for yaw rate control. With a CVT on both of the half shafts, independent torque can be applied to each wheel without having a motor on each of the wheels.

Conventional torque vectoring method using front wheel torque is also simulated, which comes out with the best results.